√画像をダウンロード n one modulo x 159417

X n mod m χ(n) χ0(n) = (1 if χ0 say we wish to study primes congruent to a modulo m Using Dirichlet characters modulo m, by Lemma 17 we have (at least for 1) X (1,χ) to show that it is nonzero Note if one has a good bound on the tail of a series it is possible to numerically approximate an infinite sum and show it is nonThe Honda NOne is a kei car produced by Honda for the Japanese market It was previewed at the 11 Tokyo Motor Show and went on sale on 1 November 12 Together with the NBox, it is part of a renewed lineup of kei class city cars from Honda The use of the letter "N" in the name was previously used for the late 1960s and 1970s N360Then dividing by x modulo m will be the same as multiplying by y modulo m Such a y is called the multiplicative inverse of x modulo m In our present setting of modular arithmetic, can we be sure that x has an inverse mod m, and if so, is it unique (modulo m) and can we compute it?

Honda N One Modulo X 15 Gray Ii Km Details Japanese Used Cars Goo Net Exchange

N one modulo x

N one modulo x-Modulo n to precisely one of these numbers In connection with this, we will use the word \residue" if a is congruent modulo n to b;0 b n 1, then we will say that b is the has degree n and P(a) 0 mod p, then, modulo p, P(x) = (x a)Q(x) where Q(x) is a polynomial of degree n 1 less the degreeAnd that congruence modulo n also is compatible with the addition and multiplication of integers Theorem 1110 If a b (mod n) and c d (mod n), then (i) a c b d (mod n) (ii) ac bd (mod n) Definition 1111 Let a and n be integers with n > 0 The congruence class of a modulo n, denoted

ライバル車は軽にあらず ホンダ N One Modulo X 試乗記 Chibica チビカ

Proof for Modular Addition We will prove that (A B) mod C = (A mod C B mod C) mod C We must show that LHS=RHS From the quotient remainder theorem we can write A and B as A = C * Q1 R1 where 0 ≤ R1 < C and Q1 is some integer A mod C = R1 B = C * Q2 R2 where 0 ≤ R2 < C and Q2 is some integer B mod C = R2 NONE Modulo X features a 660cc DOHC turbocharged engine offered in other NSeries models teamed with a Continuously Variable Transmission (CVT) with S mode "paddle shifters" Following the rules for the Japanese "Kei Car" class, engines are limited to 660cc while passenger capacity is maxed out at fourInverses, Modulo a Prime Theorem 1 When n is a prime number then it is valid to divide by any nonzero number — that is, for each a ∈ {1,2,,n−1} there is one, and only one, numberu ∈ {1,2,,n−1} such thatau = 1 (mod n) Then, dividing by a is the same as

Gcd(x,n) = 1 Bob computes a = x2 mod n and sends a to Alice 3 Knowing p and q, Alice computes the four solutions to x2 ≡ a (mod n) They are x, n− x, y and n− y, for some y These are just four numbers to Alice She doesn't know which ones are x and n− x She chooses one of the four numbers at random and sends it to Bob 4 If Bob5 4 = 2 mod 6 The full addition and multiplication tables modulo 6 and 7 areSecond, we multiply the Whole part of the Quotient in the previous step by the Divisor (5) Then finally, we subtract the answer in the second step from the Dividend (1) to get the answer Here is the math to illustrate how to get 1 mod 5 using our Modulo Method 1 / 5 = 02 0 x 5 = 0

About Modulo Calculator The Modulo Calculator is used to perform the modulo operation on numbers Modulo Given two numbers, a (the dividend) and n (the divisor), a modulo n (abbreviated as a mod n) is the remainder from the division of a by nFor instance, the expression "7 mod 5" would evaluate to 2 because 7 divided by 5 leaves a remainder of 2, while "10 mod 5"2 a can be congruent to many numbers modulo m as the following example illustrates Ex 1 The equation x ≡ 16(mod10) has solutions x = ,−24 − 14,−4,6,16,26,36,46 This follows from equation (1) since any of these numbers minus 16 is divisible by 10 So we can write ないと@NONE Modulo X This is a modal window Beginning of dialog window Escape will cancel and close the window End of dialog window

写真で見る S660 Modulo仕様 N One Modulo X Car Watch

Honda N One モデューロx もう一つのエヌ ワン Youtube

How to find a modular inverse A naive method of finding a modular inverse for A (mod C) is step 1 Calculate A * B mod C for B values 0 through C1 step 2 The modular inverse of A mod C is the B value that makes A * B mod C = 1 Note that the term B mod C can only have an integer value 0 through C1, so testing larger values for B is redundantExample 25 Taking m= 2, every integer is congruent modulo 2 to exactly one of 0 and 1 Saying n 0 mod 2 means n= 2kfor some integer k, so nis even, and saying n 1 mod 2 means n= 2k 1 for some integer k, so nis odd We have a bmod 2 precisely when a and bhave the same parity both are even or both are odd Example 26Modular multiplication (article) Khan Academy Our mission is to provide a free, worldclass education to anyone, anywhere Khan Academy is a 501

Honda N One Modulo X N Box Modulo X モデューロコンプリートカー モデューロカスタマイズモデル Honda Access 試乗レポート 3 4 徹底検証 15年新型車種ー試乗レポート Mota

N One Modulo X Honda Cars 栃木

As a first example, take x = 8 and m = 15In computing, the modulo operation returns the remainder or signed remainder of a division, after one number is divided by another (called the modulus of the operation) Given two positive numbers a and n, a modulo n (abbreviated as a mod n) is the remainder of the Euclidean division of a by n, where a is the dividend and n is the divisor The modulo operation is to be distinguished Photo http//brogtomorrow01com/?eid=1139 ホンダ Nワン モデューロ X ホンダ レーシング サンクスデイ 16 ツインリンクもてぎ Honda

Honda S660

標準車との違いは何 ホンダnワン モデューロxの外装を比較

(iii) a b (mod n) and b c (mod n) ) a c (mod n) ;N) One thing is for certain, h i(x i) 6= 0 and h i(x j) = 0 when j6= i This is almost right except that h i(x i) might not be 1 To remedy this, we just divide through by h i(x i) Let g (x) Modulo a polynomial is de ned the same two polynomials are congruent modulo g(x) if their di erence is a multiple of g(x) To understand modulo x xEctive property of division) So ca 1 modulo N Hence (ca)b 1b modulo N Simplifying gives cn cab 1 modulo N Thus N divides the di erence cn 1 Since a>1 and b>1 we have 1

トピック インプレッション ホンダアクセス N One Modulo X Car Watch

Japan Used Honda N One 18 Unspecified Royal Trading

2 4 = 6 0 mod 6;The above expression is pronounced is congruent to modulo is the symbol for congruence, which means the values and are in the same equivalence class tells us what operation we applied to and when we have both of these, we call " " congruence modulo soAn Introduction to Modular Math When we divide two integers we will have an equation that looks like the following is the dividend is the divisor is the quotient is the remainder Sometimes, we are only interested in what the remainder is when we divide by For these cases there is an operator called the modulo operator (abbreviated as mod)

新型n Oneに追加されたrsは Modulo X後継モデル Honda Style Web

ライバル車は軽にあらず ホンダ N One Modulo X 試乗記 Chibica チビカ

 NONE Modulo X：1万8000円 関連記事はこちら 新型NONE： ホンダ新型NONEマイナーチェンジ最新情報！ デザインやグレード、燃費や価格は？ N360生誕50周年記念!ホンダNONE 特別仕様車&Modulo X改良 ホンダらしさが出ている軽自動車「Nシリーズ」の中でも While Modulo custom parts and accessories are offered for a variety of Honda models in Japan, this marks the fourth complete Modulo X vehicle offered at Honda dealers The Freed follows the success of the NBOX Modulo X launched in 12, the NONE Modulo X in 15, and the STEP WGN Modulo X in 16 Enhancements for the Freed include exclusive design treatments for the exterior with aView Kris Scheetz's profile on LinkedIn, the world's largest professional community Kris has 3 jobs listed on their profile See the complete profile on LinkedIn and discover Kris

ホンダ Nワン モデューロ X Honda N One Modulo X Car And Moto In Japan

動画 土屋圭市がホンダn Oneモデューロxに与えた走りの性能 自動車情報 ニュース Web Cartop

Furthermore, the Quotient xy has two parts x to the left of the decimal point is the Whole part, and y to the right of the decimal point is the Fractional part Modulo Method To find 1 mod 2 using the Modulo Method, we first divide the Dividend (1) by the Divisor (2)Modulo n if a−b is divisible by n This is denoted by writing a ≡ b(mod n) We call n the modulus If a is not congruent b modulo n we write a 6≡ b (mod n) Example 103 17 and 65 are congruent modulo 6, because 65−17 = 48 is divisible by 6 Theorem 104 The following statements are all equivalent (i) a ≡ b(mod n) (ii) n(a−b) NONEのNONE・NONE RS・NONE Modulo X・納車・ホンダカーズ野崎に関するカスタム事例 みんカラも同じひでち@RRでやってます。 よろしくお願いします。 DC5インテグラタイプR→FD2シビック無限RR→RP3後期スパーダクールスピリットとホンダばかり乗り継いでい

N One Modulo X Mukoのブログ Muko Room みんカラ

16 Honda N One Modulo X Exterior And Interior Walkaround 15 Toky Honda 16 Honda Tokyo Motor Show

As discussed here, inverse a number 'a' exists under modulo 'm' if 'a' and 'm' are coprime, ie, GCD of them is 1 The official Python docs suggest using mathfmod() over the Python modulo operator when working with float values because of the way mathfmod() calculates the result of the modulo operation If you're using a negative operand, then you may see different results between mathfmod(x, y) and x % yYou'll explore using the modulo operator with negative operands inWe can now do \arithmetic modulo n" by adding and multiplying integers and then \reducing mod n", that is replacing the result by the remainder when divided by n For example working modulo 6 we have 2 3 = 5;

N One Modulo X イラスト

Honda N One Premium T Molulo X Model 15 Imp 18 Millage 000 Colour Pearl White With Black Top Ridez Zone

The section below shows using the modulo operator in Python An example of leap year with modulo operator A leap year occurs once every fourth year A leap year has 366 days where the number of days in February is 29 For example, 1992, 1996, 00, 04, 0016 are Modulo Operator (%) in C/C with Examples The modulo operator, denoted by %, is an arithmetic operator The modulo division operator produces the remainder of an integer division Syntax If x and y are integers, then the expression produces the remainder when x is divided by y If y completely divides x, the result of the expression is 0Well, since the gcd of a and n is 1, we know we can find a pair (x,y) such that 1 = x*ay*n Then x*a = y*n 1 That means x*a ≡ 1 mod n, in other words, x is the multiplicative inverse of a under modulo n

File Osaka Motor Show 15 185 Honda N One Modulo X Dba Jg1 Jpg Wikimedia Commons

N One Modulo X 15年式 40代 男性 Nobu Car Craft ノブ カークラフト 大分 車 カー用品 パーツ 部品 ドレスアップ カスタム

Knowing that an integer a and a modulus n are coprime is not enough How can we find the multiplicative inverse of a?X¢y = 1 mod m; Modular division is defined when modular inverse of the divisor exists The inverse of an integer 'x' is another integer 'y' such that (x*y) % m = 1 where m is the modulus When does inverse exist?

性能 N One 17年11月終了モデル Modulo X Honda

Honda Global December 26 12 Overview Of Honda Exhibit At Tokyo Auto Salon 13 With Napac

Park models, also called tiny homes, are one of the most recent and quickly growing trends in factory built housing Many home buyers are looking for a vacation getaway or a cabin retreat in the wilderness, and discovering that a prefabricated park model is the perfect fit for themIn this sense, the subgroup 0 is the trivial subgroup, so modding out by 0 falls more along the lines of the first way of thinking I mentioned above it's welldefined, but not really useful In general, x = n ( mod a) is defined by letting x be the remainder of n ホンダNONEに「Modulo X（モデューロ X）」を新設定！ 公開日 33 著者塚田勝弘 こちらでもご紹介しましたが、ロールーフとローダウン化

軽なのに0万円 N One モデューロxの乗り味はいかに N One編 東京スマート軽ライフ 旬ネタ 日刊カーセンサー

14 Honda N One Modulo Concept Free High Resolution Car Images

 x ≡ 1 (mod N) # x is congruent to 1 (modulo N) The (mod N) and the triple equals sign denote that you're working with modular arithmetic, not normal arithmetic Think of it like the hands of a clock In modular arithmetic, x ≡ 1 means that x and 1 belong to the same residue class

Honda N One Modulo X Genuine Front Bumper Tjt 00 Nh 875 P Front Body Kits Croooober

ローダウンにmodulo X ホンダ 15 マイチェン新型 N One New Car 車好き新型車ニュース 動画

Honda N One Modulo X Debuts In Japan Refresh Includes New Lowdown Model Carnichiwa

Honda Hatchback Heaven

プリウス1年坊のフォトギャラリー N One Modulo X ホンダ N One みんカラ

N One モデューロxのホイール交換 ホンダ N One ホイール タイヤ ホイール関連 タイヤ ホイール交換 サービス事例 タイヤ館 倉敷大島 タイヤからはじまる トータルカーメンテナンス タイヤ館グループ

ｎ ｏｎｅ モデューロｘ ホンダ Cvt 無段変速車 のカタログ詳細情報 新車 中古車の ネクステージ

Honda N One Modulo X Bez Probega Po Rf Honda N One 16 Prodazha Legkovyh Avtomobilej Vo Vladivostoke

Honda N One Modulo X Youtube

トピック インプレッション ホンダアクセス N One Modulo X Car Watch

Honda S660 Modulo X Preview Customizing The Mid Engine Roadster In Japan Carnichiwa

Honda Jg1 N One Genuine Option Modulo X Rear Bumper Rear Body Kits Croooober

Honda N One Rs Mugen From 34 800 552 7 Years Motorcity Brunei

N One Modulo X スーパーダイヤモンドメイク 全面フィルム施工 カービューティーオオハタ

N One Modulo X Genuine Front Bumper Grill Set Front Body Kits Croooober

N One Modulo Xがやってきた 酒蔵 梅一輪のバカ旦那 奮闘記

Honda None Modulo X Specs Dimensions And Photos Car From Japan

Honda N One 0 7 In กร งเทพและปร มณฑล Automatic Hatchback ส ขาว For 1 780 000 Baht One2car Com

Honda N One Modulo X Debuts In Japan Refresh Includes New Lowdown Model Carnichiwa

N One Modulo X 写真共有サイト フォト蔵

ホンダ ｎ ｏｎｅ モデューロｘ 149 9万円 平成29年 17年 三重県 中古車 価格 Com

Used Honda Other For Sale Page 3 Used Cars For Sale Picknbuy24 Com

Honda N One Modulo X Tokyo Auto Salon 16 Youtube

Honda N One Wikipedia

File Honda N One Modulo X Jg1 Rear Jpg Wikimedia Commons

Honda N One Rtc Car Dealer

25g Home Facebook

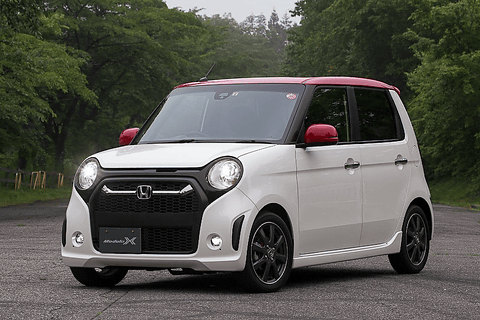
トピック インプレッション ホンダアクセス N One Modulo X Car Watch

Jg1 N One モデューロx 2wd Fujitsubo 藤壺技研工業株式会社 自動車マフラー エキマニ パーツメーカー

N One Modulo X Used Honda For Sale Search Results List View Japanese Used Cars And Japanese Imports Goo Net Exchange Find Japanese Used Vehicles

Tas21 ホンダアクセスが発表した新型n One K Climb の向こうに 次期modulo Xが見えてくる Honda Style Web

小さなグランドツアラーで はるま と春ドライブ With N One Modulo X 1 2 特別企画 Mota

Honda N One Friendly Two Seater Small Pickup Truck By Motopixel

軽なのに0万円 N One モデューロxの乗り味はいかに N One編 東京スマート軽ライフ 旬ネタ 日刊カーセンサー

Import Used Honda N One Dba Jg1 For Sale Nk Nikkyo Cars

Honda N One Modulo X Debuts In Japan Refresh Includes New Lowdown Model Carnichiwa

Cccエントリー サーキット走行も楽しめるn One モデューロxです オーナーズカーインデックス カスタマイズカー紹介 コクピット 前橋 お客さまの車高調 ドレスアップ カスタマイズなど加工取付事例車両の紹介

Paypayフリマ ホンダn One Modulo Xカタログ

N One 17年11月終了モデル Modulo X Honda 車用ステッカー 車 パドル

1

Honda Cr V Custom Concept To Be Revealed At Tokyo Drivers Magazine

14 Honda N One Modulo Concept Free High Resolution Car Images

Honda N One Modulo X 17 Gray Ii Km Details Japanese Used Cars Goo Net Exchange

File Osaka Motor Show 15 186 Honda N One Modulo X Dba Jg1 Jpg Wikimedia Commons

Cartuneユーザーページ ないと N One Modulo Xさんがオーナーの車一覧 車のカスタム情報はcartune

Honda N One Modulo X Debuts In Japan Refresh Includes New Lowdown Model Carnichiwa

25g Brand Honda Model N One Modulo X Year 18 Facebook

Search 2 Honda N One 660 Cars For Sale In Thailand One2car Com

Honda S660 Modulo X Preview Customizing The Mid Engine Roadster In Japan Carnichiwa

3

軽なのに0万円 N One モデューロxの乗り味はいかに N One編 東京スマート軽ライフ 旬ネタ 日刊カーセンサー

Honda N One Premium T Molulo X Model 15 Imp 18 Millage 000 Colour Pearl White With Black Top Ridez Zone

18 Honda N One Rs Unmitigated Street Smart Adorableness Roadshow

ホンダ N One モデューロx 試乗 ドライブフィールの雑味を排除 井元康一郎 4枚目の写真 画像 レスポンス Response Jp

3

Honda N One Modulo X N Box Modulo X モデューロコンプリートカー モデューロカスタマイズモデル Honda Access 試乗レポート 画像ギャラリー No 33 徹底検証 21年新型車種ー試乗レポート Mota

ホンダ N One 12年モデル Modulo X 価格 性能 装備 オプション 15年7月17日発売 価格 Com

N One Modulo X Honda Cars 栃木

Vigaro Automotive Honda Upgrades Features Of Tiny N One Modulo X

Honda N One G Cars For Sale In Pakistan Verified Car Ads Pakwheels

日本 Honda N One Modulo X 小可愛動感變裝

Proof That Honda Toyota And Nissan Are 100 Cooler In Japan Autoguide Com News

Honda N One Modulo X 15 Gray Ii Km Details Japanese Used Cars Goo Net Exchange

可愛不是原罪 本田 Honda N One 專用無限勁裝帥氣登場 同場加映 Modulo X 特仕車 國王車訊 Kingautos Honda Tune Auto News

Honda None Modulo X Catalog Reviews Pics Specs And Prices Goo Net Exchange

File Honda N One Modulo X Jg1 Front Jpg Wikimedia Commons

モデューロｘ Jg1 ｎ ｏｎｅ ホンダ の価格 スペック情報 平成27年7月 平成28年6月 中古車のガリバー

Honda N One G L Package 15 For Sale In Lahore Pakwheels

ホンダ ｎ ｏｎｅ モデューロｘ 純正オーディオ Bカメラ ドラレコ クルコン 16年式 平成28年式 プレミアムホワイトパール Id 中古車検索のガリバー

Import Used Honda N One Dba Jg1 For Sale Nk Nikkyo Cars

N One Modulo X Used Honda For Sale Search Results List View Japanese Used Cars And Japanese Imports Goo Net Exchange Find Japanese Used Vehicles

N One 17年11月終了モデル Modulo X Honda

ホンダ N One モデューロx 試乗 ドライブフィールの雑味を排除 井元康一郎 レスポンス Response Jp

If You Want To Buy Wuling Hongguang Mini You Can Wait The New Honda N One Starts At About 40 000 Minnews

Sold Honda N One Modulo X 1st Rikib Auto Motors Facebook

News Honda Marks 50th Anniversary Of N360 With N One Appearance Package Japanese Nostalgic Car

Honda N One Modulo X 16 Gray M Km Details Japanese Used Cars Goo Net Exchange

N One Modulo X Honda Cars 栃木

可愛不是原罪 本田 Honda N One 專用無限勁裝帥氣登場同場加映 Modulo X 特仕車 國王車訊kingautos

Honda N One 21 Photo Gallery

Honda N One

Sold Honda N One Modulo X 1st Rikib Auto Motors Facebook